Crystal Classes – Geology Notes – For W.B.C.S. Examination.
স্ফটিক গত্রর ধরন – ভূতত্ত্ব নোট – WBCS পরীক্ষা।
The discipline of crystallography has developed a descriptive terminology which is applied to crystals and crystal features in order to describe their structure, symmetry, and shape. This terminology defines the crystal lattice which provides a mineral with its ordered internal structure. It also describes various types of symmetry. By considering what type of symmetry a mineral species possesses, the species may be categorized as a member of one of six crystal systems and one of thirty-two crystal classes.Continue Reading Crystal Classes – Geology Notes – For W.B.C.S. Examination.
The concept of symmetry describes the periodic repetition of structural features. Two general types of symmetry exist. These include translational symmetry and point symmetry. Translational symmetry describes the periodic repetition of a motif across a length or through an area or volume. Point symmetry, on the other hand, describes the periodic repetition of a motif around a point.
Reflection, rotation, inversion, and rotoinversion are all point symmetry operations. A reflection occurs when a motif on one side of a plane passing through the center of a crystal is the mirror image of a motif which appears on the other side of the plane. The motif is said to be reflected across the mirror plane which divides the crystal. Rotational symmetry arises when a structural element is rotated a fixed number of degrees about a central point before it is repeated. If a crystal possesses inversion symmetry, then every line drawn through the center of the crystal will connect two identical features on opposite sides of the crystal. Rotoinversion is a compound symmetry operation which is produced by performing a rotation followed by an inversion.
A specified motif which is translated linearly and repeated many times will produce a lattice. A lattice is an array of points which define a repeated spatial entity called a unit cell. The unit cell of a lattice is the smallest unit which can be repeated in three dimensions in order to construct the lattice. The corners of the unit cell serve as points which are repeated to form the lattice array; these points are termed lattice points.
The number of possible lattices is limited. In the plane only five different lattices may be produced by translation. The French crystallographer Auguste Bravais (1811-1863) established that in three-dimensional space only fourteen different lattices may be constructed. These fourteen different lattice structures are thus termed the Bravais lattices.
The reflection, rotation, inversion, and rotoinversion symmetry operations may be combined in a variety of different ways. There are thirty-two possible unique combinations of symmetry operations. Minerals possessing the different combinations are therefore categorized as members of thirty-two crystal classes; each crystal class corresponds to a unique set of symmetry operations. Each of the crystal classes is named according to the variant of a crystal form which it displays. Each crystal class is grouped as one of the six different crystal systems according to which characteristic symmetry operation it possesses.
A crystal form is a set of planar faces which are geometrically equivalent and whose spatial positions are related to one another by a specified set of symmetry operations. If one face of a crystal form is defined, the specified set of point symmetry operations will determine all of the other faces of the crystal form.
A simple crystal may consist of only a single crystal form. A more complicated crystal may be a combination of several different forms. The crystal forms of the five non-isometric crystal systems are the monohedron or pedion, parallelohedron or pinacoid, dihedron, or dome and sphenoid, disphenoid, prism, pyramid, dipyramid, trapezohedron, scalenohedron, rhombohedron and tetrahedron. Fifteen different forms are possible within the isometric system.
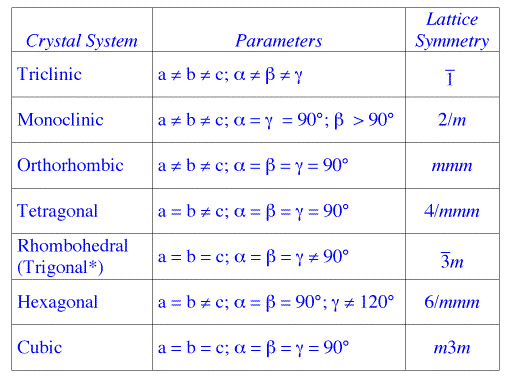
Each crystal class is a member of one of six crystal systems. These systems include the isometric, hexagonal, tetragonal, orthorhombic, monoclinic, and triclinic systems. The hexagonal crystal system is further broken down into the hexagonal and rhombohedral divisions. Every crystal of a certain crystal system will share a characteristic symmetry element with the other members of its system. The crystal system of a mineral species may sometimes be determined visually by examining a particularly well-formed crystal of the species.
Symmetry
Crystals possess a regular, repetitive internal structure. The concept of symmetry describes the repetition of structural features. Crystals therefore possess symmetry, and much of the discipline of crystallography is concerned with describing and cataloging different types of symmetry.
Two general types of symmetry exist. These consist of translational symmetry and point symmetry. Translational symmetry describes the periodic repetition of a structural feature across a length or through an area or volume. Point symmetry, on the other hand, describes the periodic repetition of a structural feature around a point. Reflection, rotation, and inversion are all point symmetries.
Lattices
The concept of a lattice is directly related to the idea of translational symmetry. A lattice is a network or array composed of single motif which has been translated and repeated at fixed intervals throughout space. For example, a square which is translated and repeated many times across the plane will produce a planar square lattice.
The unit cell of a lattice is the smallest unit which can be repeated in three dimensions in order to construct the lattice. In a crystal, the unit cell consists of a specific group of atoms which are bonded to one another in a set geometrical arrangement. This unit and its constituent atoms are then repeated over and over in order to construct the crystal lattice. The surroundings in any given direction of one corner of a unit cell must be identical to the surroundings in the same direction of all the other corners. The corners of the unit cell therefore serve as points which are repeated to form a lattice array; these points are termed lattice points. The vectors which connect a straight line of equivalent lattice points and delineate the edges of the unit cell are known as the crystallographic axes.
The number of possible lattices is limited. In the plane only five different lattices may be produced by translation. One of these lattices possesses a square unit cell while another possesses a rectangular unit cell. The third possible planar lattice possesses a centered rectangular unit cell, which contains a lattice point in the center as well as lattice points on the corners. The unit cell of the fourth possible planar lattice is a parallelogram, and that of the final planar lattice is a hexagonal unit cell which may alternately be considered a rhombus.
Please subscribe here to get all future updates on this post/page/category/website


 +919674493673
+919674493673  mailus@wbcsmadeeasy.in
mailus@wbcsmadeeasy.in